Elastic-hardening

The initial slope is defined by the elastic modulus, $E$, after yield the hardening modulus $E_h$ governs as stress rises from $(\varepsilon_y,f_{yd})$ to $(\varepsilon_u,f_{u})$. For EN 1992 the hardening modulus is defined in terms of a hardening coefficient $k$ and the final point is $(\varepsilon_{uk},kf_{yd})$ where the failure strain is reduced to $\varepsilon_{ud}$ (typically $0.9\varepsilon_{uk}$).

The relationship between hardening modulus and hardening coefficient is:

$$E_h\frac{(k-1)f_y}{\varepsilon_{uk}-f_y/E}$$

$$k=\frac{E_h(\varepsilon_{uk}-f_y/E)}{f_y}+1$$

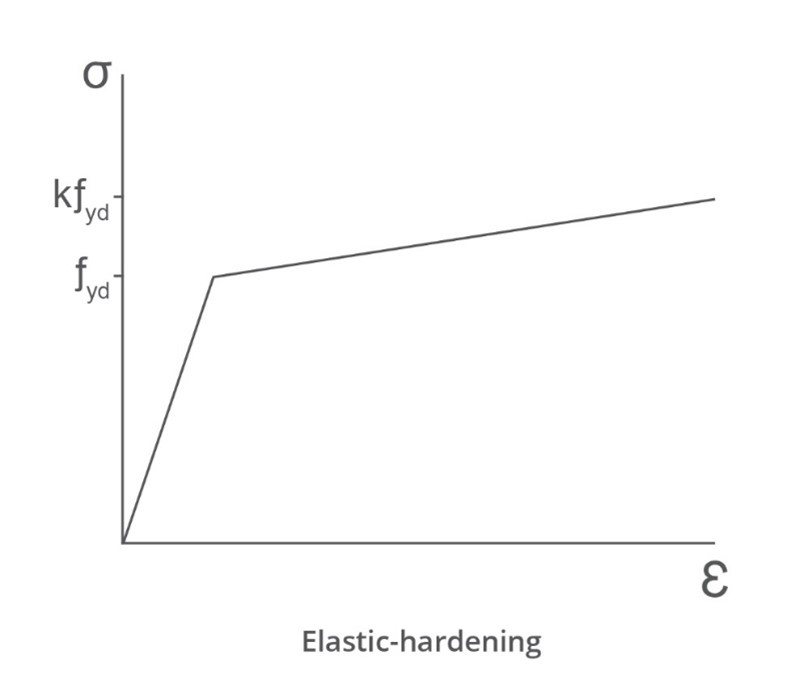
The material fails at $\varepsilon_{ud}$ where $\varepsilon_{ud}<\varepsilon_{uk}$. This is defined in Eurocode and related codes.