Rectangular Sections
The torsion constant J is given by
J=Kb3bmax where K is a constant depending on the ratio of bbmax,
which can be read either from the table below. Linear interpolation may
be used for intermediate values.
| bbmax | K | | bbmax | K | | bbmax | K | | bbmax | K |
|---|
| 1.0 | 0.141 | | 1.5 | 0.196 | | 2.8 | 0.258 | | 10.0 | 0.312 |
| 1.1 | 0.154 | | 1.8 | 0.216 | | 3.0 | 0.263 | | ∞ | 0.333 |
| 1.2 | 0.166 | | 2.0 | 0.229 | | 4.0 | 0.281 | | | |
| 1.3 | 0.175 | | 2.3 | 0.242 | | 5.0 | 0.291 | | | |
| 1.4 | 0.186 | | 2.5 | 0.249 | | 7.5 | 0.305 | | | |
or from the graph
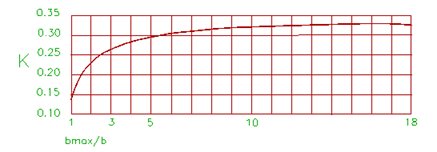
Note that K converges to 1⁄3 for narrow rectangles.
Alternatively
K=31[1−0.63bmaxb(1−12bmax4b4)] generally, or if bmax>2b then
(1−12bmax4b4)≈1 and so
K=31[1−0.63bmaxb] 